Declination of the Sun
"It's gonna be a bright, bright sun-shiny day!"
Words to Begin With
- Horizon is the horizontal plane that extends from the
point where the observer is standing, to infinity, straight
through space. Since we're only working with relatively short
distances (compared to the Universe), a line extending N-S will
be quite sufficient.
- Altitude (A) is the angle of the sun over the horizon. In
this problem, we will be working with the sun at noon, so it
will either be over the N or S horizon.
- Zenith (Z) is the angle that the sun is from directly
overhead, and it is equal to 90-A. It, too, can be over the
S or N horizon, but there is little need to state it.
- Declination (D) is the latitude at which the sun is directly
overhead. It is always between 23.5 N and 23.5 S latitude,
those occurring on the Solstices.
- Latitude (L) is the location N or S of the equator at which
the observer is located. (It is determined by radii from the
center of Earth at different angles to the equator. If such
an angle is swept along the surface of the planet, it draws
a circle.)
A Quick Lesson in Basic Geometry
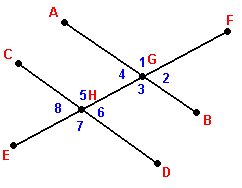
Diagram A
In Diagram A above, CD and AB are parallel, intersecting EF at G and H,
respectively. Congruent (same) sets of angles are: 1, 3, 5, and 7; and
2, 4, 6, and 8. This is because opposite angles (ie: 1 and 3) are
congruent (which is true for any 2 intersecting lines), and because
same-side angles of parallel lines (ie: 3 and 7) are congruent.
I will refer to this as the SS (Same-Side) theorem.
And the Rest is Simple Mathematics...
Honest, I'm not kidding about this! I couldn't do these for the
longest time, but I finally figured out how and where I went wrong:
I was trying to make these problems a lot harder than they were!
Just relax; they aren't that hard. Take it from someone who knows.
Three Possible Cases
Important Note: If you are given information about D, and you
know either L or A, you can get whichever of them you
do not have.

Case 1.
Case 1.
- Z as the sum of D and L, from the SS Theorem.
- Z will therefore be larger than both D and L.
- D and the observer are in different hemispheres.

Case 2.
Case 2.
- L is the sum of D and Z, from the SS theorem.
- L is greater than each D and Z.
- D and the observer are in the same hemisphere.
- The observer is farther from the equator than D is.
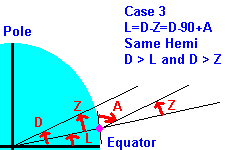
Case 3.
Case 3.
- D is the sum of L and Z, from the SS theorem.
- D is greater than each L and Z.
- D and the observer are in the same hemisphere.
- The observer is closer to the equator than D is.
How 'Bout an Example?
Suppose it is the first day of summer, and the sun is over 23.5 N, and
you've just observed the sun at an altitude of 50 degrees over the
S horizon. What's your latitude?
First, let's examine what we have as information.
- Declination = 23.5 N.
- Altitude = 50 over S horizon.
Next, what can we infer?
- Zenith = 40.
- Since we have to look S to see the sun with a declination over
a line of N latitude, we must be further to the N than the
declination, and we are in the same hemisphere as the
declination.
- This is, therefore, a Case 2 situation.
Now, we solve for our latitude.
- D + Z = L; 23.5 + 40 = 63.5 N (remember we said it is in
the same hemisphere as the declination.)




